Mass-Spring System

A mass-spring system is shown below:

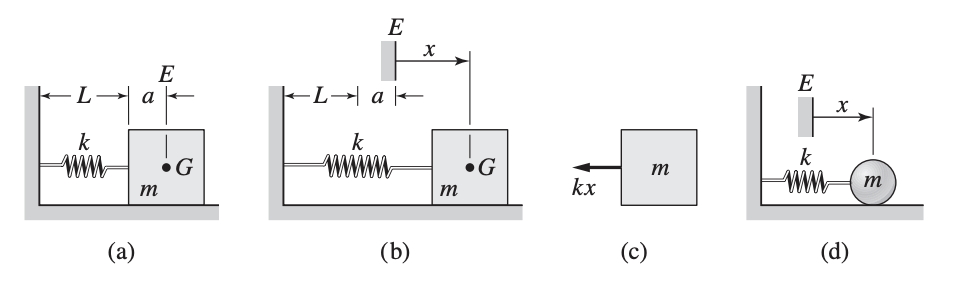
Newton’s 2nd law in combination with Hooke’s Law:

$$\begin{array}{rl}f=m\frac{dv}{dt}& =m\frac{d^{2}x}{d{t}^2}=m\ddot{x}\\ -kx& =m\ddot{x}\text{or}m\ddot{x}+kx=0\end{array}$$

The mass is at equilibrium when the spring is at its free length ($x=0$).

Equilibrium as Coordinate Reference

If we write the equation of motion based on the equilibrium position as the coordinate reference, the equation of spring systems will be $m\ddot{x}+kx=0$.

Inclined Plane Example

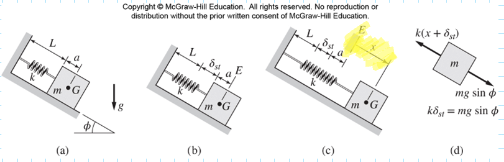
For a static deflection ${\delta }_{st}$ at equilibrium position, we would have:

$$\begin{array}{rl}k{\delta }_{st}-mg\sin \varphi & =0\\ k{\delta }_{st}& =mg\sin \varphi \end{array}$$

In the above example, we have:

$$\begin{array}{rl}m\ddot{x}& =-k({\delta }_{\text{st}}+x)+mg\sin \varphi \\ m\ddot{x}& =-kx+\underset{0}{\underbrace{(mg\sin \varphi -k{\delta }_{\text{st}})}}\\ m\ddot{x}& =-kx\end{array}$$

The term on the right side will be 0 at static equilibrium.

Vertical Spring Example

In the example above, we have:

$$\begin{array}{rl}m\ddot{x}& =-k({\delta }_{x+\text{st}})+mg\\ & =-kx+\underset{0}{\underbrace{(mg-k{\delta }_{\text{st}})}}\end{array}$$

where $mg-k{\delta }_{\text{st}}=0$ if the system is static.

Frequency

For a mass-spring system, the frequency of oscillation can be found as:

$${\omega }_n^2=\frac{K}{m}$$

Solving for $\omega$ gives:

$${\omega }_n=\sqrt{\frac{K}{m}}$$

Converting to frequency form:

$$2\pi f_n=\sqrt{\frac{K}{m}}$$

or

$$f_n=\frac{1}{2\pi }\sqrt{\frac{K}{m}}$$