Electromechanical Systems

See also:

• AC generator
• DC motor

The majority of electromechanical devices utilize a magnetic field. Two basic principles apply to a conductor, such as a wire, carrying a current within a magnetic field:

1. A force is exerted on the conductor by the field and
2. If the conductor moves relative to the field, the field induces a voltage in the conductor that opposes the voltage producing the current.

In many applications, the following model relates the force $f$ to the current $i$:

$$f=BLi$$

where $B$ is the flux density in $\text{Wb}/{\text{m}}^2$ and $L$ is the length of the conductor. The direction of the force, which is perpendicular to the conductor and the field, can be found with the right-hand rule. This equation is valid for two situations:

1. Straight conductors that are perpendicular to a uniform magnetic field
2. Circular conductors in a radial field

When the directions of the field, the conductor, and its velocity are mutually perpendicular, the second principle can be expressed as:

$$v_b=BLv$$

where $v_b$ is the voltage induced in the conductor by its velocity $v$ in the field. Again using the right-hand rule, we find the positive direction of the current induced by $v_b$ by sweeping the fingers from the positive direction of v to the positive direction of the field.

The two principles and the expressions can be represented graphically as in Figure 6.5.1.

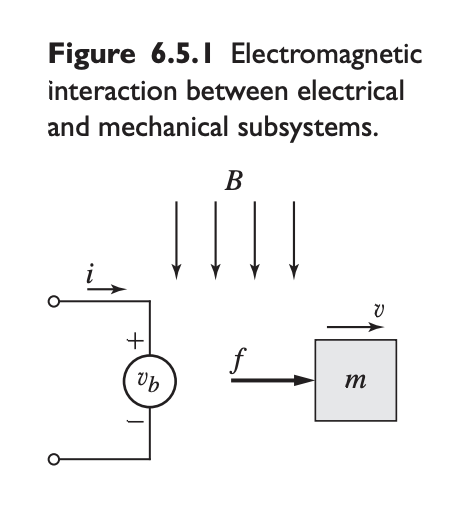
• The circuit represents the electrical behavior of the conductor and the mass $m$ represents the mass of the conductor and any attached mass.
• The power generated by the circuit is $v_{b}i$. The power applied to the mass $m$ by the force $f$ is f v. Neglecting any energy loss due to resistance in the conductor or friction or damping acting on the mass, we see that no power will be lost between the electrical subsystem and the mechanical subsystem, and thus,