Root Locus Design for Double Integrator System

Consider a double integrator with a $P$-gain:

The closed-loop transfer function is:

$$\frac{\frac{K}{s^2}}{1+\frac{K}{s^2}}=\frac{K}{s^2+K}$$

The characteristic equation is:

$$s^2+K=0$$

which means the closed poles are at $s=\pm \sqrt{K}j$.

This would result in:

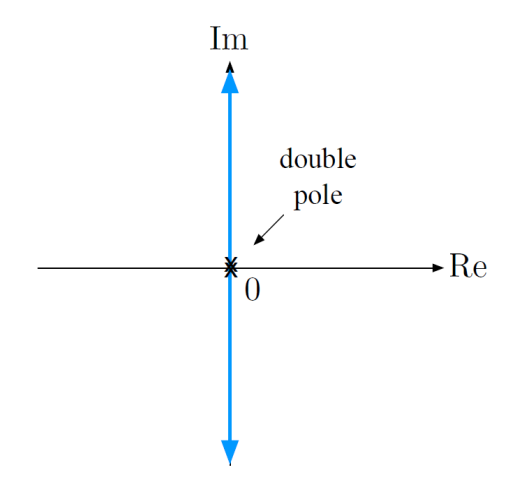
• This confirms what we already knew – $P$-gain alone does not deliver stability.

Now consider using a PD controller instead:

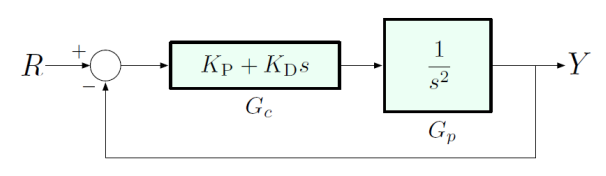
Characteristic equation:

$$\begin{array}{r}1+(K_P+K_{D}s)\cdot \frac{1}{s^2}=0\\ s^2+K_{D}s+K_P=0\end{array}$$

We need to convert this into the Evans form of $1+KL(s)=0$:

$$\begin{array}{r}1+(K_P+K_{D}s)\frac{1}{s^2}=0\\ 1+K_D\cdot \frac{s+K_P/K_D}{s^2}=0\end{array}$$

which lets us have $K=K_D$ and $L(s)=\frac{s+K_P/K_D}{s^2}$. Assume that $K_P/K_D$ is fixed at 1.

Then, we have a characteristic equation of

$$1+K\cdot \frac{s+1}{s^2}=0$$

Let’s draw the root locus using the rules:

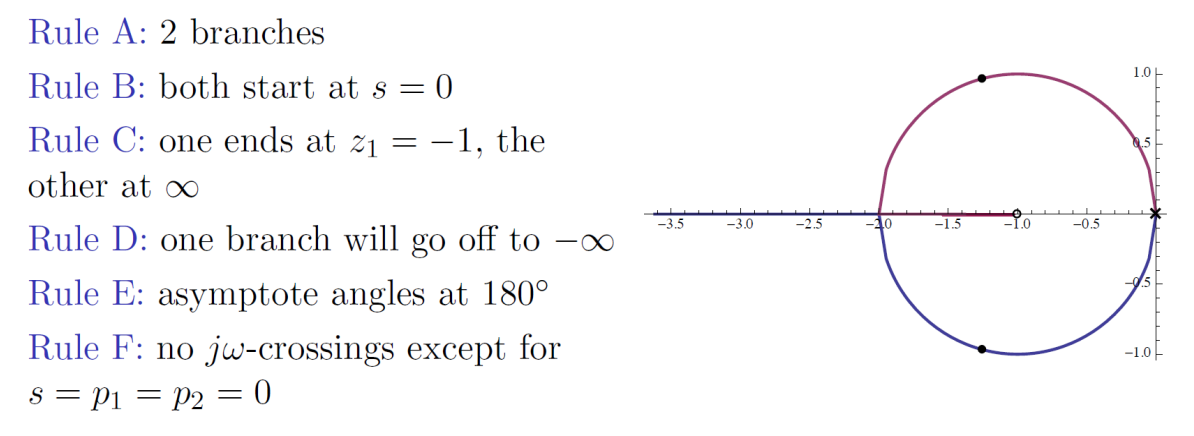
What can we conclude from this root locus about stabilization?

• All closed-loop poles are in LHP (we already knew this from Routh, but now we can visualize)
• Nice damping, so we can meet reasonable specs.