For a finite set , let denote the number of elements in . The table below compares finite sets with finite-dimensional vector spaces, showing the analogy between for sets and for vector spaces, as well as the analogy between unions of subsets (in the context of sets) and sums of subspaces (in the context of vector spaces).
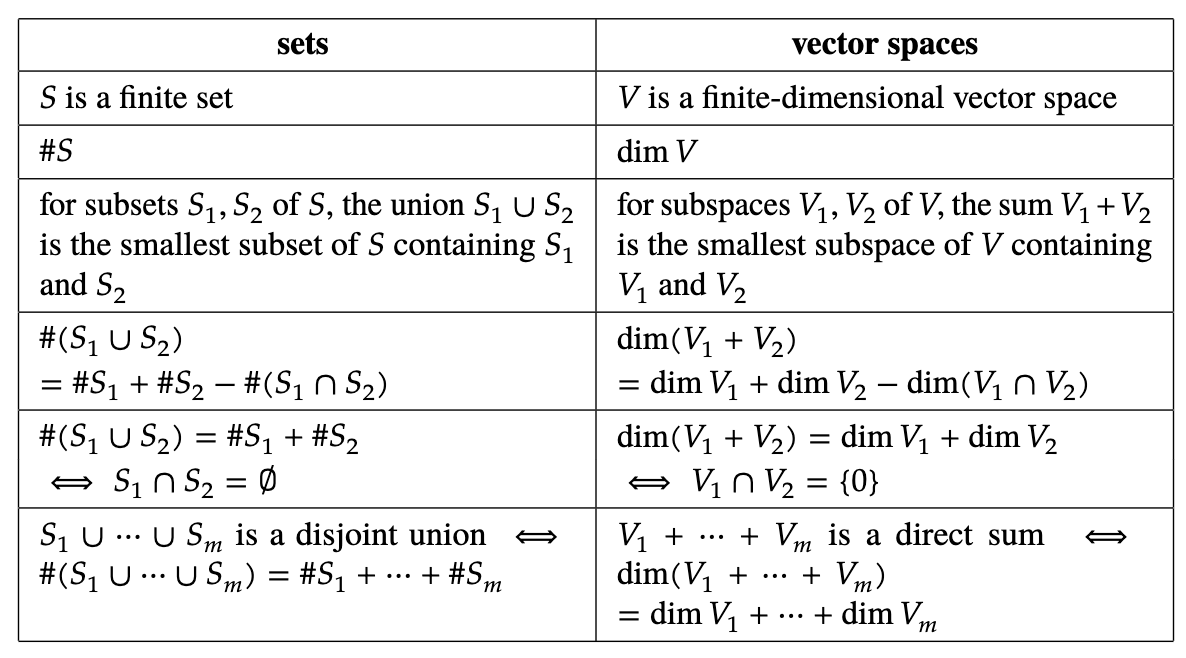
The last row focuses on the analogy between disjoint unions (for sets) and direct sums (for vector spaces). The proof of the result in the last box is given in A Sum is A Direct Sum Iff Dimensions Add Up.